Instructions for Computing the Flux Density
Litzopt uses magnetic field data to assess winding losses. The data used
is the square of the magnetic flux density (B) integrated over the volume
of each of the windings in the transformer. These values are to be
computed for all combinations of one- and two-winding excitations in the
transformer. The excitation sequence is generated by Litzopt for
any given number of windings. (see a Litzopt
data file for an example of the sequence of winding excitations).
Obtaining the Integral of Squared Flux Density
Users with access to numerical electromagnetic analysis software (e.g.
Maxwell)
can obtain the necessary field values by constructing the transformer winding
window and running a simplified "magnetostatic" simulation. The required
magnetostatic field simulation requires far less computation time than
a conventional field simulation and all that is to be computed is the spatial
integral of squared flux density over the regions of uniform winding density.
The simulation can be performed in two or three dimensions. Three-dimensional
simulations can accommodate any core geometry but are less computationally
efficient. Two-dimensional simulations offer greater computational
efficiency and can be used to obtain an approximation of the magnetic flux
density (B) of a three-dimensional device. More information
regarding the use of two-dimensional simulations to model a three-dimensional
geometry is available here.
For a given winding excitation, all of which consist of unit current
(NI = 1 ampere-turn) in one winding or unit currents in two windings considered
simultaneously, the volume integral of the squared field value considered
over a winding is defined as the spatial integral of the squared field
everywhere within the volume occupied by the winding. For a
given excitation, this calculation is to be repeated over the volume of
each of the windings in the device. When the volume integral of squared
field has been obtained in each transformer winding, the user is to apply
current through the winding(s) excited in the next winding excitation sequence
in the data file.
Three-Dimensional Simulations
Users simulating in three dimensions are to draw the transformer and obtain
the integral of squared flux density over each of the regions specified
in the winding excitation sequence. The user is to draw the transformer
and follow the sequence of excitations with a total NI of 1 ampere-turn
in the excited windings (corresponding to a winding current of I
= 1 ampere/N ).
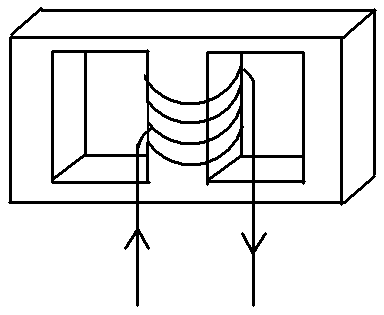
A three-dimensional drawing of an ecore-transformer
Two-Dimensional Simulations
Two-dimensional simulations are often used to obtain approximations of
magnetic fields in three-dimensional devices. Most core geometries
can be accommodated using two-dimensional solutions. Unfortunately,
the following two-dimensional simulation procedures cannot accommodate
all core geometries (e.g. toroidal). Users simulating in two dimensions
are to draw the transformer by 'slicing' through the center and drawing
the resulting cross section (see example below). The windings' cross
sections are to be considered as regions of uniform current and so each
winding cross section is to be drawn as a rectangle. The user is
to obtain the volume integral of squared magnetic flux by multiplying the
area integral of squared flux density over the winding cross-section from
the two-dimensional simulation by the average length of a winding turn
(2
r); to be
computed for each winding (see
example). An alternate method of obtaining the volume integral
of squared magnetic flux is by performing a RZ (cylindrical) simulation
by integrating over an incremental volume of 2r
dA with the integrand being the quantity B2
dA. The resulting integral values are to be multiplied by the
factor 2to obtain
the volume integral over each of the winding regions.
A cross-section of a typical winding window. The windings are
in
blue and are considered as regions of uniform current density.